As often happens, I'll publish an initial post on some subject, then add bells and whistles on to my code, then post the "finished" product with a few quips, and expect the audience to have insight into the chaos of my workflow.
So I've decided to put the wind model down on blog-paper, at least to make it clearer to myself and others. I was inspired by
Here Dragons Abound, who also revisited his wind model and arrived at the same method that I did (just with better pictures)! So this post is essentially the same, just with some different words and pictures, for my own reference.
Getting the initial wind values at the coast was the
easy part, really. But what to do once I have them?
Ideally, I could use some kind of iterative finite element method to solve for the flow across the surface. But that's pretty complicated and if I can find something simpler, I will.
There are a few things to keep in mind as we create this model.
- Wind slows as it drags across the ground
- Wind picks up speed when going downhill and slows uphill
- Wind is turned aside by mountains
So there are (in the most simple representation) 3 parameters that can be tuned. I'll call them $g$ for ground drag, $s$ for slope change (where $\Delta$ can represent the different in height of the hexes), and $\theta(\rho, \Delta)$ for the change in direction by a mountain (or hill, etc), where $\rho$ is the sensitivity of the wind.
Let's begin with a single wind vector.
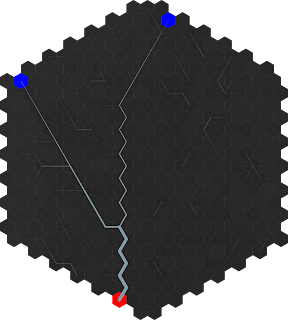
The basic method of propagation is along the vector projection of the direction between the two hexes. I refer you to this diagram from the
post on tectonics. We are interested in the $d_{\perp f}$ vector.
Projecting the vectors:
One downside of this approach is that eventually, every initial vector gets binned into one of the 6 hex directions, so the wind map becomes much more West Marches-esque, with strictly defined angles in increments of 60 degrees. But let's propagate each of these three resultants.
Next, we add the vectors (simply adding the $x$ and $y$ components) for E and B.
And of course, E and B will project onto D, and so on. But I'll ignore that for now.
So what about mountains, or other obstacles? How much is it affected? Let us assume that A represents a high mountain at 10,000 ft, and that the other hexes are 1 ft. We can then define $\theta$ in terms of $\Delta$ as:
\[\theta = {90 \exp\left(\rho\Delta\right) \over 90 + \left(\exp\left(\rho\Delta\right) - 1\right)}\]
The value $\rho={\ln(89) \over 5000}$ indicates that the wind is turned aside 45$^\circ$ at a slope of 5000 ft. So for our Mountain A, it will be 89$^\circ$. I have no idea what number will work best here. The relative direction of change is to the right in the northern hemisphere, and to the left in the southern hemisphere.
And so it continues (I haven't actually propagated A out fully).
As far as picking up speed goes, I have $s = g$ if $\Delta < 0$ else $1$. But I want to do some tweaking of this anyway (I also see a lot of stuff that I want to update/clean up, just from going through this exercise). I've been using $g = 0.946$ after my initial experiments.
So the final model for the effect of wind from Hex 0 to Hex 1 (where $h_{01}$ represents the vector from 0 to 1):
\[w_{01} = g \cdot s \cdot {w_0 \cdot h_{01} \over |h_{01}|^2} h_{01} + \angle \theta(\rho, \Delta)\]
I guess that looks kinda complicated. If you can't wow them with facts, dazzle them with bullshit.
There is also a choice to be made in the algorithm for propagating vectors. Either we can view this as an expanding frontier, where the next set of hexes to be solved is defined by where the vectors in the current frontier are pointing (projection is only ever positive for 3 hexes at most), or we can fully propagate each vector from each source (which are all coastal hexes) and then average them at the end. Right now I'm using source-propagation since its a little faster, but I'd like to take another look at frontier-propagation.
I'll save the good stuff (the actual application on the map) for another post.